Gerrymandering
How it works
Gerrymandering is the process of drawing electoral districts with the goal of maximizing the number of districts where a majority of voters favor a certain political party. Gerrymandering can lead to a scenario in which a majority of districts elect representatives from a certain political party even though a majority of voters favor the other party.
Given a map — a grid with squares colored either red or blue (with more red squares than blue, let’s say) — can you figure out how to gerrymander it so that a majority of districts have a majority of blue squares?
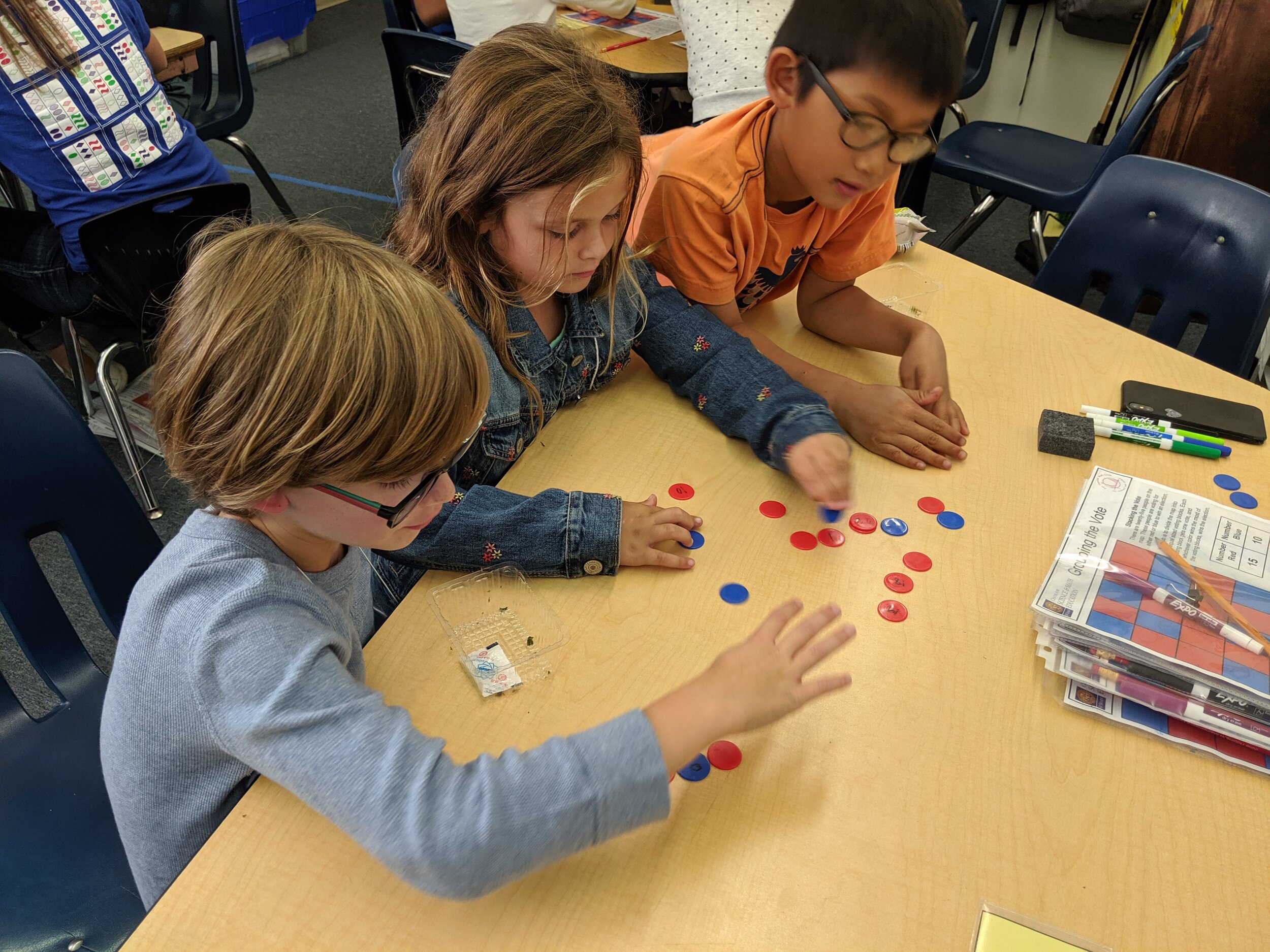
In this activity, students start by trying to group 25 chips — 15 red, 10 blue — into 5 piles with 5 chips each so that a majority of piles have a majority of blue chips (that is, so at least 3 out of 5 piles have at least 3 blue chips). They then try to figure out the minimum number of blue chips they need in order to be able to do this, and explore the same question for different numbers of piles and different numbers of chips per pile.
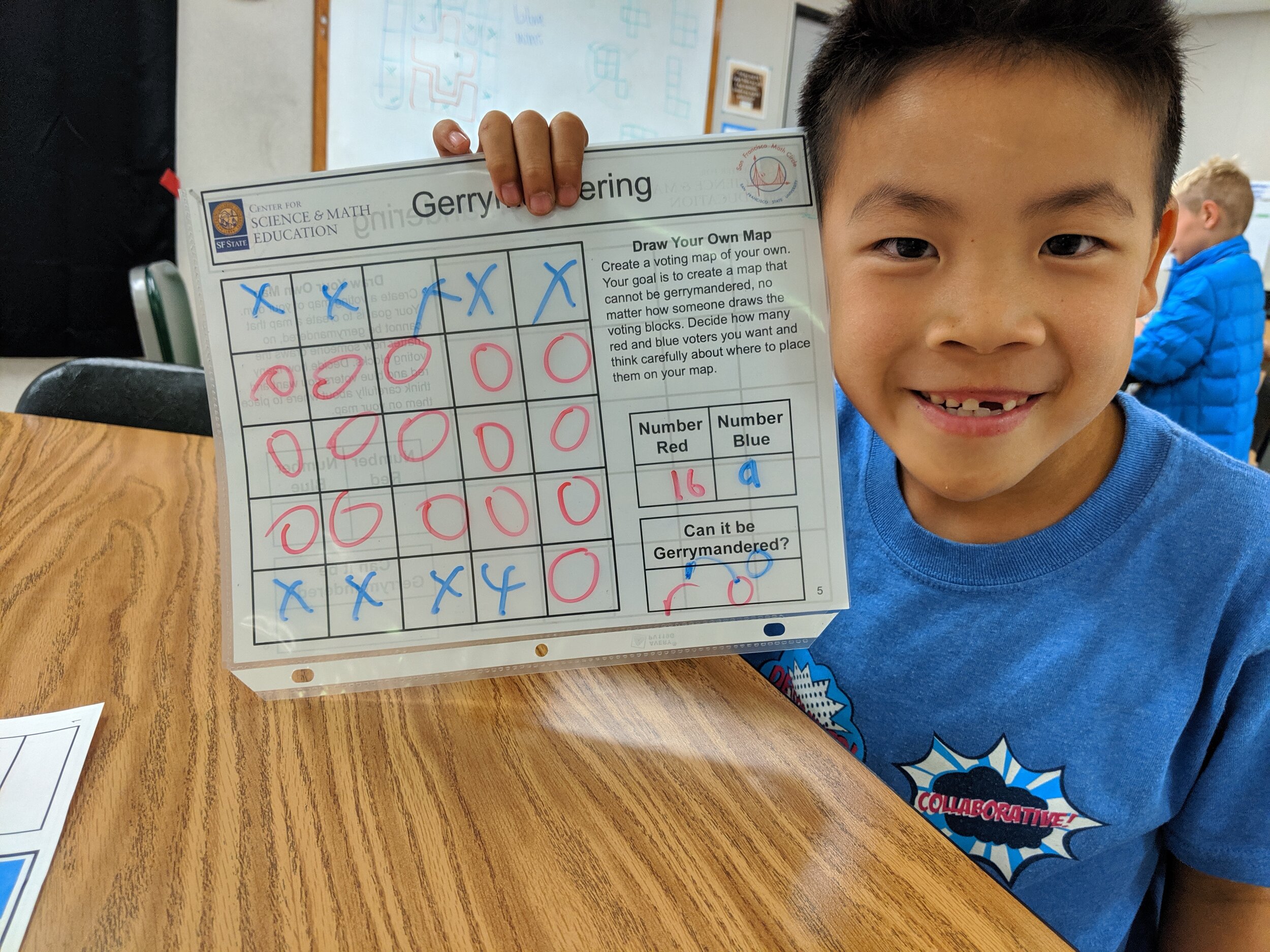
Next, they try to divide 5 x 5 square grids into 5 regions with 5 squares each so that a majority of regions have a majority of blue squares. They attempt a variety of challenge grids — some of which are possible and some of which aren't — and then they design their own challenges and explore how many blue squares they can fit into a grid while still making it impossible to gerrymander.
Gerrymandering Challenges handout
Why we like this activity
- It’s fun! Students enjoy finding ways to gerrymander the grids and designing their own grids.
- It helps students develop numerical reasoning.
- It helps students develop spatial reasoning.
- It requires students to engage in mathematical habits of mind:
- Finding and using strategies to group chips into piles so blue wins more piles than red even though there are fewer blue chips.
- Understanding and explaining why you need at least a specific number of blue chips in order for blue to win more piles than red.
- Finding and using strategies to gerrymander grids — that is, to divide them into regions so blue wins more regions than red even though there are fewer blue squares.
- Understanding and explaining why certain grids can't be gerrymandered.
- Finding and using strategies to design gerrymander-proof grids with as many blue squares as possible.
- It has a low floor and a high ceiling: Students can get started drawing regions by trial and error, but more complicated grids require more advanced strategies.